viewerzuloo.blogg.se

at a shallow angle of incidence) you will clearly see the reflection of the surroundings and sky, and little or no detail of the bottom of the pool.

steep angle of incidence) you will see the bottom of the pool.Īs you look out across the pool (i.e. Figure 5.16: When the angle of incidence is greater than the critical angle, the light ray is reflected at the boundary of the two media and total internal. So if you stand at the edge of a pool and look down near your feet (i.e. Above 50 degrees the percentage of light that is reflected increases steeply. sketch a velocity time graph and find the acceleration, deceleration, total distance covered and its average speed. Light travelling at a water surface at angles of incidence less than 50 degrees mostly penetrate the water. If you were to draw the amount of light refracted at the boundary when light travels from air to water you would get the following graph:

(3% of the light will be reflected backwards) These two principles are apposite to all kinds of reflecting planes. The ray of incidence, ray of reflection and the normal drawn at the point of incidence, to the mirror, occurs at the same plane. (Please note: In nearly all questions in exams at basic level, the proportion of light that is reflected is not taken into account)īright beam at 0° – perfectly in line with the normal line. The law of reflection says: The angle of incidence is identical to the angle of reflection. It is just in different proportions depending on the angle. In this case, a graph drawn against angle of incidence and angle. When a ray of light travels from a fast to a slow medium, for instance air to water, at different angles of incidence (degrees to the normal) you will always get some surface boundary reflection and you will always get some refraction. According to the law of reflection, the angle of incidence equals the angle of reflection. For the first case, s polarization reflectance continues to increase at all angles of incidence but R P approaches 0 at rewster’s angle ( B tan-1n 2 /n 1) and then increases rapidly with increased AOI. Total internal reflection occurs when the angle of incidence is greater than the critical angle and.

the reflected beam is purely S polarized). For the accurate measurement of the resonance angle from an SPR curve, several fitting methods have been proposed, such as the polynomial fits11,12, centroid method6and parabolic fit 13, 14. Brewster's angle is a special incidence angle which results in P polarized being completely transmitted (i.e. TIR can occur only if material 2 has a lower refractive index than material 1 and even then the incidence angle must be larger than a so called critical angle. For convenience, the calculator also computes an average result corresponding to unpolarized light.Īdditionally, this calculator computes the refraction angle, the angle for total internal reflection (TIR) and Brewster's angle.

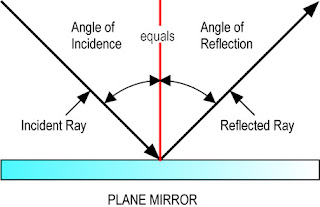
While the wave direction changes, the wave speed, wavelength and. Therefore, the results are different for S polarization (E-field vector sticking out of the paper if you draw the interface) and P polarization (E-field vector within the incidence plane). Figure 1: When a wave is reflected, the incident angle i is equal to the reflected angle r. The second law states that angle of incidence and reflection, reflected and incident ray, normal all lie in the same plane. These depend on the refractive indexes of the two materials as well as the incidence angle and polarization of the wave in material 1. is the angle between the reflected ray and the normal at the point of incidence (see Fig. According to the laws of reflection, The angle of incidence is equal to the angle of reflection and it is generally denoted as. This calculator computes the power reflectivity and transmission of a plan wave at a dielectric interface using the Fresnel equations.